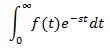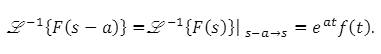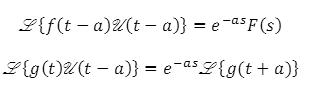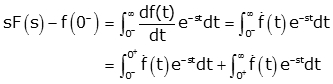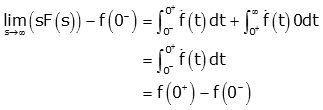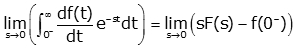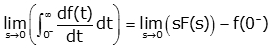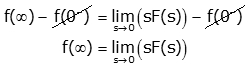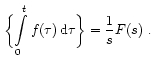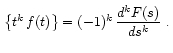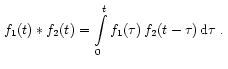Our loyal member Nasir continues his article series about Laplace Transform. Today, let him sum up some theorems…
Introduction
Laplace transform is a way of transforming differential equations into algebraic equations. The Laplace integral is given by:
As we studied Laplace transform of some basic elementary functions and properties of Laplace transform, there are a few theorems that do apply on Laplace transform and make them easier to solve.
We will also see how we can use those theorems over Laplace integral just like we did with the properties. Some of the Laplace theorems are also considered as properties so you may feel like you have studied them already in the properties article but here we shall discuss the a slightly different context.
Let’s have a look at the basic theorems:
1. Existence Theorem
The foremost theorem analysis whether or not Laplace transform of a function exists. It says that for a piecewise continuous function f (t), L (f (t)) exists if and only if t ≥ 0 and s > t.
2. The First Shifting Theorem
The first shifting theorem states that, if a function f(t) is in time domain and get multiplied by e-at, the result of s-domain shifts by amount a.
Mathematically,
3. Second Shifting Theorem
The second shifting theorem has quite similarities with the first one but the outcomes are entirely different. The unit step function converts exponential function from t-domain in s-domain. Mathematically the theorem can be stated as:
4. First Translation theorem
This theorem is applicable when a function say, f (t) is multiplied by an exponentialeat. The Laplace transform of their product is given as:
When we evaluate this integral we get the first translation theorem. So we have:
Here we have replaced s by s-a in F(s).
Inverse Form of the First Translation Theorem
Since we know that,
The inverse of the theorem is given as:
Since this is inversed form so, here we have replace s-a by s.
5. Second Translation Theorem
Consider a function f (t-a) and a unit step function u (t-a), the transform of their product is given as e-asF(s). Similarly for another function g (t) and the same unit step function u (t-a), the transform of their product is given as e-asL {g (t+a)}.
Mathematically, second translation theorem is represented as:
Inverse Form of the Second Translation Theorem
To find the inverse of second translation theorem, take the inverse of above equation:
6. Initial Value Theorem
This theorem we also discussed in properties. The theorem for initial value is
Or
To prove this theorem, we will take derivative rule:
We then evoke the Laplace transform definition and breakup integral in two parts
Taking limit s going to infinity
On left hand side, the second term doesn’t depend on s and can be pulled out. On the right hand side, the first term can be pulled out giving same justification. Also if infinity is to be put for s in second term then the exponential term goes to zero.
f (0-) is canceled from both sides and only initial value theorem remains.
7. Final Value theorem
You may remember that we discussed this theorem in properties. Unlike initial value theorem, in final value theorem only final value of function remains
Or
The limitation of this theorem is that it can be used only when final value if it exists, like for functions cosine, sine and ramp function there is no final values. To show the final value theorem, we will start with Laplace transform derivative
Taking limit s goes to zero,
For s→0, the exponential term dies out from the integral. In addition we can pull out f (0-) from limit as it is not dependent on s.
We can calculate integral
In the left hand expression, there is no term which depends on s so we can take off limit. By simplifying we will get final value theorem
8. Superposition theorem
If we take a1 and a2 as constants then theorem will be
9. Complex Shifting Theorem
Taking a>0 as an arbitrary constant
10. Similarity Theorem
For an arbitrary constant a>0
11. Derivative theorem
Taking f(t) as casual function of time, for which the derivative exists if t>0
12. Integral theorem
Function’s integral is represented by
13. Complex differentiation theorem
By this theorem, it is explained that a differentiation of a function F(s) in time domain corresponds to a multiplication with time t.
14. Convolution in the time domain
If taking two functions f1 (t)and f2 (t) which is represented by symbolization f1 (t) * f2 (t), the theorem will be
Convolution of two functions gives the multiplication of those functions, that is
That’s all about the theorems of Laplace Transform. In the coming articles, we are going to discuss the inverse Laplace Transform which has its own importance in determining the stability of any function.
Nasir.