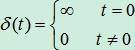Let Nasir tell you about first order systems and their response…
Just like him, you can publish an article on the blog, all you have to do is to tell us by mail. You can write about whatever you want (debate, tutorial, product review, observations, your status as an engineer or student, etc.).
Introduction
There are two methods to analyze functioning of a control system that are time domain analysis and control domain analysis. In time domain analysis the response of a system is a function of time. It analyzes the working of a dynamic control system.
This analysis can only be applied when nature of input plus mathematical model of the control system is known. It is not easy to express the actual input signals by simple equations as the input signals of the control systems are not fully known. There are two components of any system’s time response, transient response and steady response.
Typical and standard test signals are used to judge the behavior of typical test signals. The characteristics of an input signal are constant acceleration, constant velocity, a sudden change or a sudden shock. We discussed four types of test signals that are Impulse Step, Ramp, Parabolic and another important signal is sinusoidal signal. In this article we will be discussing first order systems.
First order system
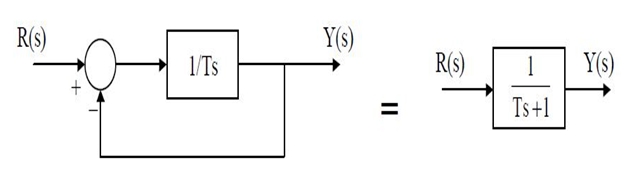
The system whose input-output equation is a first order differential equation is called first order system. The order of the differential equation is the highest degree of derivative present in an equation. First order system contains only one energy storing element. Usually a capacitor or combination of two capacitors is used for this purpose. These cannot be connected to any external energy storage element.
Most of the practical models are first order systems. If a system with higher order has a dominant first order mode it can be considered as a first order system.
Response of a first order system
It is not much difficult to find the response of a first order system as the degree of differential equation is one. There are two important points on which this analysis is actually based:
- The time constant for a first order system is given by :
t=RC (for a system with resistors and capacitors)
t=L/R (for a circuit with inductors).
- The response of a first order system is given by:
Provided that, input is constant and t>0, where v (0) is voltage or current at t=0. Now we will see the unit responses with respect to first order systems and will see the transfer functions accordingly.
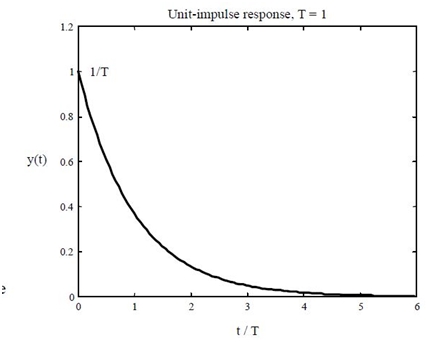
1. Unit Impulse in First Order System
- As we know the unit impulse input is: r(t) = δ(t), t ≥0
- For Laplace transform the Transfer function of input: R(s) =1.
- The output transform will be:
- By taking inverse Laplace transform: Y(t)= e-t/T/T, t ³ 0
- The impulse input generates transfer function as the output.
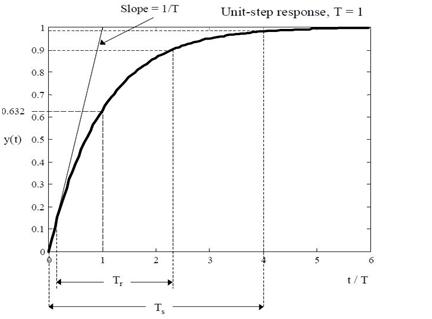
2. Unit Step Response of First Order System
- The Transfer function of input is: R(s)=1/s
- Therefore the unit step response is:
- Expanding in Partial fraction:
- Take the inverse Laplace transform: y (t) = 1 – e-t/T, t ³ 0.
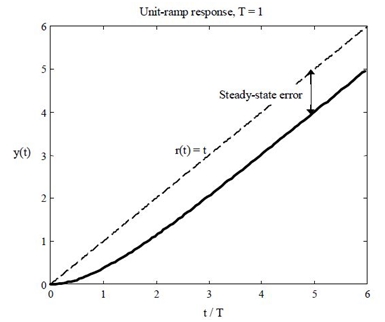
3. Unit Ramp Response of First Order System
- The input, r (t) = t for t ³ 0.
- In Laplace transform, The transfer function of input: R(s)=1/s²
- The output transform is:
- Expanding in Partial fraction:
- Taking the inverse Laplace transform: y (t) = t – T + Te-t/T, t ³ 0.
Conclusion
In this tutorial we have enlightened first order systems and their response. We calculated time constant and time response of these systems. We discussed both time response and ramp response of the first order systems.
The upcoming tutorial will discuss the same aspects of second order systems so stay tuned. We have much more to study and share with you in the upcoming articles.
Nasir.